|
|
|
|
Alternate View Column AV-78
Keywords: particle physics
standard model top quark lepton mass force coupling strength alternate universe
Published
in the May-1996 issue of Analog Science Fiction & Fact
Magazine;
This column was written and submitted 10/24/95 and is
copyrighted ©1995 by John G. Cramer.
All rights reserved. No part may be
reproduced in any form without
the explicit permission of the author.
The hot news in particle physics last year was the March 2, 1995 announcement that the top quark (or Top) had been discovered and its mass measured by the CDF and D0 proton-antiproton collider experiments at Fermilab. The Top had been a missing piece in the particle physics jigsaw puzzle. The Top, the heaviest of the six quarks of the Standard Model, had been predicted and its discovery expected for several years, but the measured size of the Top mass came as a big surprise.
Before the Fermilab measurements, the "smart money" was betting that the Top would have a mass around 120 GeV. (Here using E = mc2, we give mass in energy units. For reference, a proton has a mass of 0.938 GeV.) The Fermilab measurements indicated a Top mass of about 180 GeV. Theorists are still agonizing over the meaning and implications of this larger-than-expected mass for the heaviest quark of our universe.
But the question raised repeatedly in the news media was: What difference
does it make? Who cares if the Top mass is 180 GeV or 120 GeV? What
possible effect could it have on the "real world" of Medicare and rock stars
and ethnic cleansing and Superbowls and insider trading? In this column we
will present some ideas from a colloquium given recently at the University of
Washington by Dr. Robert N. Cahn of Lawrence Berkeley National Laboratory that address these questions. We'll start by considering the Standard Model of
particle physics.
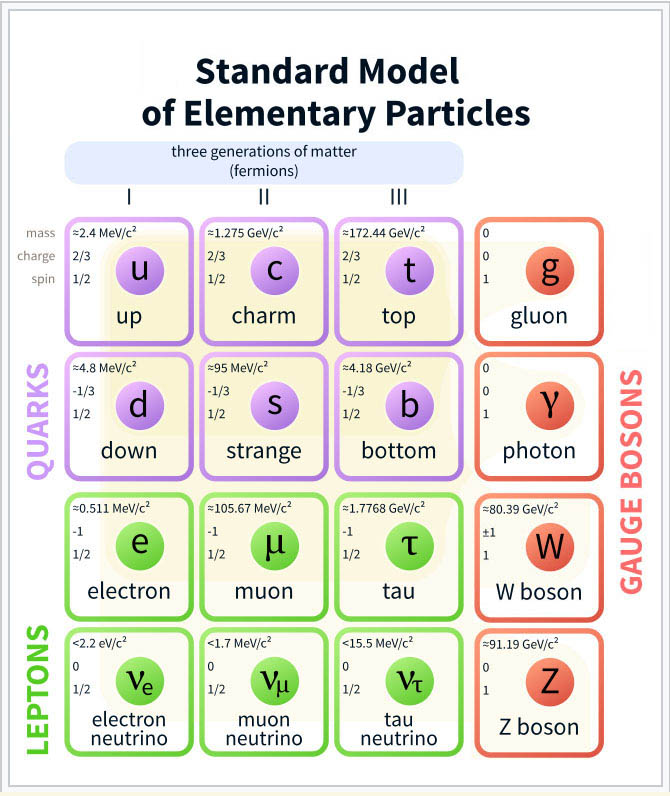
The Standard Model is the physical theory that embodies our current understanding of the microcosm. It uses as particle building blocks the six quarks (down, up, strange, charmed, bottom, and top), the six leptons (electron, mu, tau, and their respective neutrinos), and the mediating particles that generate the four forces of nature. There are 14 mediating particles: eight color-carrying gluons for the strong force, one photon for the electromagnetic force, three "left-handed weakons" W+, W-, and Zo for the weak force, and (perhaps) one graviton for the gravitational force. The Standard Model describes the interactions between these particles in terms of four constants which characterize the strengths of the forces and four coupling constants which indicate the ease with which one quark can be converted to another by the weak force. In addition, it describes the "unification" of the strong and electro-weak interaction at sufficiently high energy and invokes a massive Higgs boson and a related strength constant to accomplish this.
The Standard Model is very frustrating for those of us who want to gain a deeper understanding of the universe by finding places where our current understanding fails. On the one hand, the Standard Model provides remarkable predictive power. At this writing there are no experimental results in serious conflict with its predictions. On the other hand, the Standard Model is based on the values of about two dozen adjustable parameters: the particle masses and the strength and coupling constants. If we ignore gravitation and set all neutrino masses and "right-handed" weak interactions to zero, there are 18 remaining parameters. We have no real understanding of where these 18 numbers come from or how they might be interconnected. We are stuck in this present state of knowledge because we have not yet found a way to "break" the Standard Model (as relativistic and quantum effects "broke" Newtonian mechanics) so that we can gain understanding of underlying mechanisms.
Nevertheless, the predictive power of the Standard Model permits us to explore
how the universe might be different if the parameter values were changed. This
was done by in his colloquium by Robert Cahn. It is an exercise that gives
interesting insights into how the real world would be different if the
parameters of the Standard Model had different values. We'll present some of
Cahn's scenarios here.
§ Scenario 1 - Increase mup:
In the Standard
Model, the down and up quarks have masses of about 10 MeV and 5 MeV,
respectively, and the mass of the neutron (2 down quarks plus 1 up quark)
exceeds the mass of the proton (1 down quark plus 2 up quarks) by 1.3 MeV.
Suppose we increase the up quark mass by 2.6 MeV, so that the proton becomes
heavier than the neutron by 1.3 MeV. What kind of universe would
result?
If the proton were heavier than the neutron, all free protons in the universe would become radioactive and would decay into neutrons by the emission of a neutrino and a positron. The normal matter of the universe would consist of neutrons, electrons, and positrons.
The gravitational attraction responsible for the formation of stars and galaxies in our universe would still be in effect, but the stars that formed would be neutron stars from the outset and would have qualitatively different stellar dynamics. Fusion, combining four neutrons to form a helium nucleus would still take place, so the neutron stars would be luminous. However, since no electrical repulsion would inhibit the fusion process it would proceed much more rapidly, and much smaller stellar masses would be able to achieve fusion and become luminous, and these also would burn out and produce supernovas more rapidly.
The synthesis of heavier elements, if there were no blockages arising from the
altered masses and level structures of nuclei, would perhaps be possible, but
the "valley of stability" of the resulting elements and isotopes would be
shifted toward more neutron-rich elements. For example, carbon-14 would become
a stable isotope, which the result that nitrogen-14 would be radioactive. It
is questionable if life could exist in such a universe. At minimum, the "real
world" in that universe would be very different from our present one.
§ Scenario 2 - Increase me:
In the Standard
Model there are three generations of charged leptons, the electron (me
= 0.511 MeV), the muon (mmu = 106 MeV),and that tau
(mtau = 1,784 MeV). Suppose that the muon was the lightest charged
lepton (or equivalently, that the electron's mass was 207 times larger so that
it had the mass of a muon). What kind of universe would result?
First, all atoms would be 207 times smaller because the Bohr radius of an atomic orbit is inversely proportional to the electron mass. Second, electrons would be bound to atoms 207 times more strongly because of the smaller orbits, and the photons produced when an electron moves from one atomic orbit to another would be more energetic by a factor of 207. Visible light from atomic transitions would be shifted into the X-ray region. Third, the time scale for atomic processes would also be increased by factor of 207. However, the speed of light itself would be unchanged, so atoms would show more effects of relativity.
One might think that in this heavy-electron universe would be a nice compact environment. If all the atoms of our bodies were scaled down together, bio-chemistry would work as usual, and we would not even notice the change. Everything would fit into a smaller space, with lots of energy and with atomic and biological processes progressing on "fast forward". Very efficient. However, there's a hitch.
In our universe a negative muon has a high probability of being captured by an atom. If captured, it rapidly moves to the innermost atomic orbit, where its orbit brings it inside the nucleus with a high probability. In less than a microsecond the atomic nucleus captures the muon, changing a proton to a neutron in the process and spitting out a muon neutrino. The resulting nucleus has one less proton, one more neutron, and may be radioactive.
This same process would occur with the electrons in our heavy-electron
universe. Both neutrons and protons would be stable particles, but all atoms
(charged nuclei with orbiting electrons) would be unstable to electron capture
that would reduce the nuclear charge with each capture. This capture process
would cumulate in the reduction of all matter to neutrons and electrons. There
would be no stable atoms, no chemistry, no biology, and therefore no
life-as-we-know-it.
§ Scenario 3 - Only one generation:
In the Standard
Model there are three generations of leptons and three generations of quarks.
Suppose that only the "middle" generation, the muon, its neutrino, and the
strange and charmed quarks, existed. What kind of universe would result?
In the first generation of quarks in our universe, the up and down quarks have
masses that are very close in energy. This is not true for the second
generation. The charmed quark has a mass of about 1500 MeV and the strange
quark has a mass of about 150 MeV. Therefore, there will not be any stable
particles containing charmed quarks. Therefore there will be no neutron-like
or proton-like stable particles which are a combination of the two quark
flavors, and consequently no stable nuclear isotopes. The middle-generation
universe will contain only two stable particles, muons and omega- baryons
which are a combination of three strange quarks. The omega would be the only
stable nuclear-mass particle in the universe. All the atoms in this universe
will be hydrogen-like, with muons orbiting omegas. If stars form from these
mu-omega atoms, there will be no fusion or nucleosynthesis because there are no
other nuclei.
§ Scenario 4 - Decrease mtop:
The top
quark according to the recent Fermilab measurements has a mass of about 180
GeV. The other member of its generation, the bottom quark, has a mass of about
4.5 GeV. Suppose we drop the top quark mass by a factor of 10 to 18 GeV. What
kind of universe would result?
The force constants of the Standard Model are not really "constants", but rather indicate the force strengths in the low energy limit of our "real world". The strengths of the forces of nature vary with energy, and at sufficiently high energies all the forces have the same strength. Thus, the true force strengths are set at very high energies and evolve downward as the energy is dropped. The evolution of the force strengths occurs because in an interaction process more varieties of virtual particles can be created at high energies than at low. Therefore, the masses of the virtual particles affects their participation and determine the resulting force strength in the low energy limit.
Cahn calculates that if the top quark had a mass of 18 GeV instead of 180 GeV, the resulting evolution of the electromagnetic interaction would be to decrease its low energy strength by about 0.5%. All electrical forces would be weaker by one part in 200. The rate of fusion reactions in the sun is proportional to the reciprocal of the electromagnetic strength constant to the 9th power. A 0.5% decrease in electromagnetic strength would result in a 4.5% increase in energy production in the sun. Curiously, this would not increase the solar temperature but the solar radius. The sun would swell and appear slightly larger in the sky, and as a larger object at the same temperature, it would deliver more solar energy to its planets.
Cahn also showed that a Top mass of 18 GeV would reduce the strength of the strong interaction, and this would reduce the masses of the proton and neutron, because their masses primarily arise from strong interaction gluon effects rather than the up and down quark masses. He calculates that the neutron and proton, with the up and down quark masses held at their Standard Model values, would be decreased in mass to 87% of their "real world" values.
In the "real world", chemical and biological processes would be affected by a
weakening of the electromagnetic force (and therefore chemical bonding) and by
reduction in the masses of atoms. The drop in neutron and proton masses would
also effect nuclear reaction and decay processes, particularly radioactive
decay and nuclear fusion. It would shift the positions of energy levels in
nuclei, and this might inhibit the synthesis of heavy nuclei in stars. It is
very difficult to assess the overall effect of these changes, but it should be
clear that in a universe where the Top mass was a factor of 10 smaller, things
would be very different in the real world.
Our present universe, which depends in subtle but important ways on the values of the Standard Model parameters, works very well the way it is. As we have seen, most variants involving parameter changes lead to unpleasant results. By what process were the Standard Model parameters set to the values that we measure and that produce the life-sustaining "real world" in which we live?
I wish I knew.
John G. Cramer's 2016 nonfiction book (Amazon gives it 5 stars) describing his transactional interpretation of quantum mechanics, The Quantum Handshake - Entanglement, Nonlocality, and Transactions, (Springer, January-2016) is available online as a hardcover or eBook at: http://www.springer.com/gp/book/9783319246406 or https://www.amazon.com/dp/3319246402.
SF Novels by John Cramer: Printed editions of John's hard SF novels Twistor and Einstein's Bridge are available from Amazon at https://www.amazon.com/Twistor-John-Cramer/dp/048680450X and https://www.amazon.com/EINSTEINS-BRIDGE-H-John-Cramer/dp/0380975106. His new novel, Fermi's Question may be coming soon.
Alternate View Columns Online: Electronic reprints of 212 or more "The Alternate View" columns by John G. Cramer published in Analog between 1984 and the present are currently available online at: http://www.npl.washington.edu/av .
References:
Top Quark Discovery:
Vincent Kiernan, "Chicago quark hunters come out on top", New Scientist v145(n1968), p6 (March 11, 1995).
Sharon Begley, "How many scientists does it take to screw in a quark", Newsweek v123(n19), p54 (May 9, 1994).
Ray Ladbury, "Where do you go when you've made it to the top ", Physics Today v48(n5), p17 (May, 1995).
|
|
|
|
This page was created by John G. Cramer on 7/12/96.